jilowalker.blogg.se

1. #CONVERTING RECURRING DECIMALS TO FRACTIONS HOW TO#
2. #CONVERTING RECURRING DECIMALS TO FRACTIONS PROFESSIONAL#
3. #CONVERTING RECURRING DECIMALS TO FRACTIONS SERIES#

#CONVERTING RECURRING DECIMALS TO FRACTIONS PROFESSIONAL#

Tutors, instructors, experts, educators, and other professionals on the platform are independent contractors, who use their own styles, methods, and materials and create their own lesson plans based upon their experience, professional judgment, and the learners with whom they engage. Keywords: long division decimal fraction convert repeating decimal divide bar repeating. Varsity Tutors connects learners with a variety of experts and professionals. convert a fraction into the repeating decimal it represents. Varsity Tutors does not have affiliation with universities mentioned on its website. Media outlet trademarks are owned by the respective media outlets and are not affiliated with Varsity Tutors.Īward-Winning claim based on CBS Local and Houston Press awards. is equal to the fraction 6/33.Names of standardized tests are owned by the trademark holders and are not affiliated with Varsity Tutors LLC.Ĥ.9/5.0 Satisfaction Rating based upon cumulative historical session ratings through 12/31/20. Answer the questions in the spaces provided there may be more space than you need. So we can see that our original decimal of 0.18181818. Recurring Decimals to Fractions Name: Instructions Use black ink or ball-point pen. To solve for a, divide each side by 99 and then simplify: (d) Subtract Equation 3 from Equation 2, then solve for a. We won't need to modify Equation 1 since it is already in that form.

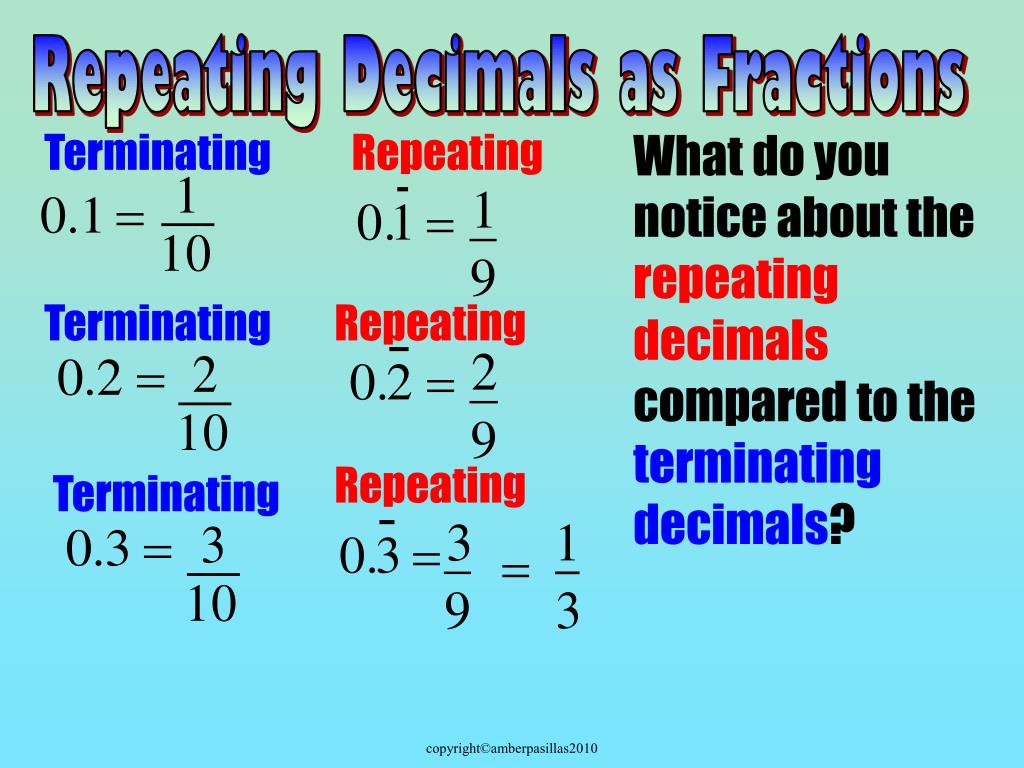
(c) We want another equation in which the repeating part is to the right of theĭecimal point. (b) Multiply both sides of Equation 1 by 100 so the "18" appears directly to the left of the decimal point. (a) The repeating part of the decimal is "18". After simplifying you will have the fraction. Sometimes Equation 1 is already in this form. This may involve multiplying by 10, 100, or another power of ten. (c) Modify Equation 1 so that the repeating part appears directly to the right This usually involves multiplying by 10, 100, or another power of ten. (b) Modify Equation 1 so that the repeating part appears directly to the left Then set the decimal equal to a variable. (a) Identify the repeating part in the decimal. Let's summarize the steps to follow to convert any repeating decimal to a fraction. So we can see that our original decimal of 0.333333. Left with 3 - 0 = 3 on the right hand side. Since the decimal part of both equations is the same, when we subtract we are just Let's subtract the first from the second. Step 4 - Notice we now have two equations. Notice that we can do this by multiplying both sides of the equation by 10. Step 3 - Modify the equation so that the repeating part (the "3") appears to the left of the decimal point. Step 2 - Set the decimal equal to some variable. Step 1 - Identify the repeating part in the decimal 0.333333. Once you know the method you can convert any repeating decimal to its equivalent You may have known that, but there is a method we can use to find the fraction. The first repeating decimal above is equal to the fraction 1/3.

#CONVERTING RECURRING DECIMALS TO FRACTIONS HOW TO#

Now let's learn how to convert a repeating decimal to a fraction. It turns out that every repeating decimal can be converted to a fraction.Īnd once it is a fraction, it is easier to use if we need to do a calculation.

#CONVERTING RECURRING DECIMALS TO FRACTIONS SERIES#

In a repeating decimal one digit, or a series of digits, keeps repeating without end. What is a repeating decimal? Here's an example: In this lesson we will learn about "repeating decimals", and how we can express them as a fraction. And the decimal 0.25 can be written as the fraction 1/4. For example, the fraction 1/2 can be written as the decimal 0.5. Students studying pre-algebra learn that fractions can be written as decimalsĪnd decimals can be written as fractions.